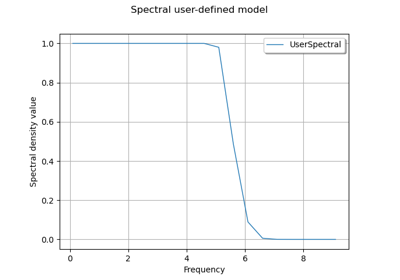
UserDefinedSpectralModel¶

(Source code, png)

class UserDefinedSpectralModel(*args)¶

Spectral model defined by the User.

Parameters:

frequencyRegularGrid: The frequency grid $(-f_c, \dots, f_c)$ with step $\delta f$ on which the model is built.
densityCollectionFunctionHermitianMatrixCollection: Collection of hermitian matrices $\in \mathbb{M}_d(\mathbb{C})$ which are the images of each point of the frequency grid through the density spectral function.

Notes

We consider a multivariate process $X: \Omega \times \cD \rightarrow \Rset^d$ of dimension $d$ where $\cD \in \Rset^n$ . We only treat here the case where the domain is of dimension 1: $\cD \in \Rset$ ( $n=1$ ).

We denote $(\vect{t}_0, \dots, \vect{t}_{N-1})$ the time stamps of the time grid.

$X$ is supposed to be a stationary second order process and we suppose that its spectral density function $S : \Rset \rightarrow \mathcal{H}^+(d)$ defined by:

(1)¶ $\forall \vect{f} \in \Rset^n, \,S(\vect{f}) = \int_{\Rset^n}\exp\left\{ -2i\pi <\vect{f},\vect{\tau}> \right\} C^{stat}(\vect{\tau})\di{\vect{\tau}}$

exists, where $\mathcal{H}^+(d) \in \mathcal{M}_d(\Cset)$ is the set of d-dimensional positive definite hermitian matrices.

We get a piecewise constant function on $[-f_c,f_c]$ , where the intervals on which the density spectral function is constant are centered on the points of the frequency grid, of length $\delta f$ .

Then, it is possible to evaluate the spectral density function for a given frequency thanks to the method computeSpectralDensity: if the frequency is not inside the interval $[-f_c,f_c]$ , OpenTURNS returns an exception. Otherwise, it returns the hermitian matrix of the subinterval of $[-f_c,f_c]$ that contains the given frequency.

Examples

Create the frequency grid:

>>> import openturns as ot
>>> from math import exp
>>> fmin = 0.1
>>> df = 0.5
>>> N = int((10.0 - fmin)/ df)
>>> myFrequencyGrid =  ot.RegularGrid(fmin, df, N)

Define the spectral function:

>>> def s(f):
...     if(f <= 5.0):
...         return 1.0
...     else:
...         x = f - 5.0
...         return exp(-2.0 * x * x)

Create the collection of HermitianMatrix:

>>> myCollection = ot.HermitianMatrixCollection()
>>> for k in range(N):
...     frequency = myFrequencyGrid.getValue(k)
...     matrix = ot.HermitianMatrix(1)
...     matrix[0, 0] = s(frequency)
...     myCollection.add(matrix)

Create the spectral model:

>>> mySpectralModel = ot.UserDefinedSpectralModel(myFrequencyGrid, myCollection)

Methods

`computeStandardRepresentative`(frequency)	Compute the standard representant of the spectral density function.
`draw`(*args)	Draw a specific component of the spectral density function.
`getAmplitude`()	Get the amplitude parameter of the spectral density function.
`getClassName`()	Accessor to the object's name.
`getFrequencyGrid`()	Return the frequency grid associated to the model.
`getInputDimension`()	Get the input dimension of the spectral density function.
`getName`()	Accessor to the object's name.
`getOutputCorrelation`()	Get the spatial correlation matrix of the spectral density function.
`getOutputDimension`()	Get the dimension of the SpectralModel.
`getScale`()	Get the scale parameter of the spectral density function.
`hasName`()	Test if the object is named.
`setAmplitude`(amplitude)	Set the amplitude parameter of the spectral density function.
`setFrequencyGrid`(frequencyGrid)	Set the frequency grid associated to the model.
`setName`(name)	Accessor to the object's name.
`setScale`(scale)	Set the scale parameter of the spectral density function.

__init__(*args)¶

computeStandardRepresentative(frequency)¶

Compute the standard representant of the spectral density function.

Parameters:

taufloat: Frequency value.

Returns:

rhoComplex: Standard representant factor of the spectral density function.

Notes

According to definitions in CovarianceModel, as the spectral density function is the Fourier transform of the stationary covariance function and using the expression of the last one, the spectral density function writes as a matrix-complex product where the matrix is the constant spatial covariance structure and the complex represents the standard representative:

Thus,

$\forall \vect{f} \in \Rset^n, \cS(\vect{f}) = \prod_{k=1}^{n} \vect{\theta}_k \mat{\Sigma} \rho(\vect{f} \odot \vect{\theta})$

where $\mat{\Sigma}$ is a covariance matrix that explains the covariance structure and $(\vect{f} \odot \vect{\theta})_k = \vect{f}_k \vect{\theta}_k$

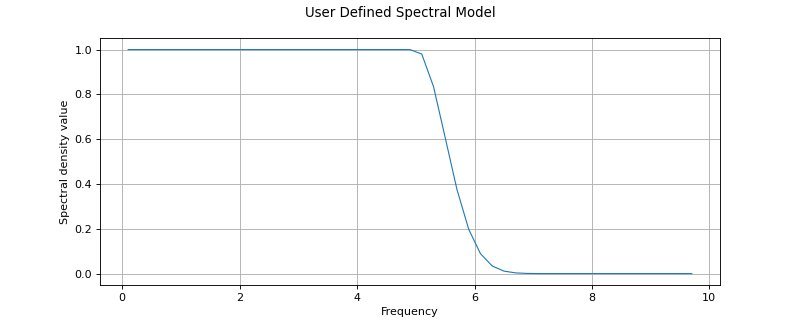
draw(*args)¶

Draw a specific component of the spectral density function.

Parameters:

rowIndexint, $0 \leq rowIndex < dimension$: The row index of the component to draw. Default value is 0.
columnIndex: int, :math:`0 leq columnIndex < dimension`: The column index of the component to draw. Default value is 0.
minimumFrequencyfloat: The lower bound of the frequency range over which the model is plotted. Default value is SpectralModel-DefaultMinimumFrequency in ResourceMap.
maximumFrequencyfloat: The upper bound of the frequency range over which the model is plotted. Default value is SpectralModel-DefaultMaximumFrequency in ResourceMap.
frequencyNumberint, $pointNumber \geq 2$: The discretization of the frequency range $[minimumFrequency, maximumFrequency]$ over which the model is plotted. Default value is SpectralModel-DefaultFrequencyNumber in class:~openturns.ResourceMap.
modulebool: Flag to tell if module has to be drawn (True) or if it is the argument to be drawn (False). Default value is True.

Returns:

graphGraph: Graphic of the specified component

getAmplitude()¶

Get the amplitude parameter of the spectral density function.

Returns:

amplitudePoint: The used amplitude parameter.

getClassName()¶

Accessor to the object’s name.

Returns:

class_namestr: The object class name (object.__class__.__name__).

getFrequencyGrid()¶

Return the frequency grid associated to the model.

Returns:

gridRegularGrid: The frequency grid of the model.

getInputDimension()¶

Get the input dimension of the spectral density function.

Returns:

inputDimensionint: SpatialDimension of the SpectralModel.

getName()¶

Accessor to the object’s name.

Returns:

namestr: The name of the object.

getOutputCorrelation()¶

Get the spatial correlation matrix of the spectral density function.

Returns:

spatialCorrelationCorrelationMatrix: Correlation matrix $\mat{R} \in \mathcal{M}_{dimension \times dimension}([-1, 1])$ .

getOutputDimension()¶

Get the dimension of the SpectralModel.

Returns:

dimensionint: Dimension of the SpectralModel.

getScale()¶

Get the scale parameter of the spectral density function.

Returns:

scalePoint: The used scale parameter.

hasName()¶

Test if the object is named.

Returns:

hasNamebool: True if the name is not empty.

setAmplitude(amplitude)¶

Set the amplitude parameter of the spectral density function.

Parameters:

amplitudePoint: The amplitude parameter to be used in the spectral density function.

setFrequencyGrid(frequencyGrid)¶

Set the frequency grid associated to the model.

Parameters:

gridRegularGrid: The frequency grid of the model.

setName(name)¶

Accessor to the object’s name.

Parameters:

namestr: The name of the object.

setScale(scale)¶

Set the scale parameter of the spectral density function.

Parameters:

scalePoint: The scale parameter to be used in the spectral density function. It should be of size dimension.

Examples using the class¶

Create a spectral model

OpenTURNS

An Open source initiative for the Treatment of Uncertainties, Risks'N Statistics

Table of Contents

Previous topic

Next topic

This Page

UserDefinedSpectralModel¶

Examples using the class¶