OptimizationProblem¶

class OptimizationProblem(*args)¶

Base class to define an optimization problem.

This represents a general optimization problem:

$\min_{x\in B} f(x) \\ g(x) = 0 \\ h(x) \ge 0$

where B is problem’s bounds, f is the objective function, g are equality constraints, and h are inequality constraints.

Available constructors:

OptimizationProblem(objective)

OptimizationProblem(objective, equality, inequality, bounds)

Parameters:

objectiveFunction: Objective function. Additional constraints and bounds must always be consistent with the objective input dimension.
equalityFunction: Equality constraints.
inequalityFunction: Inequality constraints.
boundsInterval: Bounds.

Methods

`getBounds`()	Accessor to bounds.
`getClassName`()	Accessor to the object's name.
`getDimension`()	Accessor to input dimension.
`getEqualityConstraint`()	Accessor to equality constraints.
`getId`()	Accessor to the object's id.
`getImplementation`()	Accessor to the underlying implementation.
`getInequalityConstraint`()	Accessor to inequality constraints.
`getLevelFunction`()	Accessor to level function.
`getLevelValue`()	Accessor to level value.
`getName`()	Accessor to the object's name.
`getObjective`()	Accessor to objective function.
`getResidualFunction`()	Accessor to the residual function.
`getVariablesType`()	Accessor to the variables type.
`hasBounds`()	Test whether bounds had been specified.
`hasEqualityConstraint`()	Test whether equality constraints had been specified.
`hasInequalityConstraint`()	Test whether inequality constraints had been specified.
`hasLevelFunction`()	Test whether level function had been specified.
`hasMultipleObjective`()	Test whether objective function is a scalar or vector function.
`hasResidualFunction`()	Test whether a least-square problem is defined.
`isContinuous`()	Check if the problem is continuous.
`isMinimization`([marginalIndex])	Test whether this is a minimization or maximization problem.
`setBounds`(bounds)	Accessor to bounds.
`setEqualityConstraint`(equalityConstraint)	Accessor to equality constraints.
`setInequalityConstraint`(inequalityConstraint)	Accessor to inequality constraints.
`setLevelFunction`(levelFunction)	Accessor to level function.
`setLevelValue`(levelValue)	Accessor to level value.
`setMinimization`(minimization[, marginalIndex])	Tell whether this is a minimization or maximization problem.
`setName`(name)	Accessor to the object's name.
`setObjective`(objective)	Accessor to objective function.
`setResidualFunction`(residualFunction)	Accessor to the residual function.
`setVariablesType`(variableType)	Accessor to the variables type.

Examples

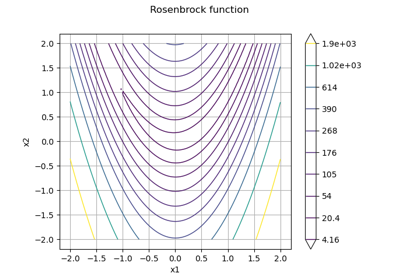
Define an optimization problem to find the minimum of the Rosenbrock function:

>>> import openturns as ot
>>> rosenbrock = ot.SymbolicFunction(['x1', 'x2'], ['(1-x1)^2+100*(x2-x1^2)^2'])
>>> problem = ot.OptimizationProblem(rosenbrock)

__init__(*args)¶

getBounds()¶

Accessor to bounds.

Returns:

boundsInterval: Problem bounds.

getClassName()¶

Accessor to the object’s name.

Returns:

class_namestr: The object class name (object.__class__.__name__).

getDimension()¶

Accessor to input dimension.

Returns:

dimensionint: Input dimension of objective function.

getEqualityConstraint()¶

Accessor to equality constraints.

Returns:

equalityFunction: Describe equality constraints.

getId()¶

Accessor to the object’s id.

Returns:

idint: Internal unique identifier.

getImplementation()¶

Accessor to the underlying implementation.

Returns:

implImplementation: A copy of the underlying implementation object.

getInequalityConstraint()¶

Accessor to inequality constraints.

Returns:

inequalityFunction: Describe inequality constraints.

getLevelFunction()¶

Accessor to level function.

Returns:

levelFunction: Level function.

getLevelValue()¶

Accessor to level value.

Returns:

valuefloat: Level value.

getName()¶

Accessor to the object’s name.

Returns:

namestr: The name of the object.

getObjective()¶

Accessor to objective function.

Returns:

objectiveFunction: Objective function.

getResidualFunction()¶

Accessor to the residual function.

Returns:

residualFunctionFunction: Residual function.

getVariablesType()¶

Accessor to the variables type.

Returns:

variablesTypeIndices: Types of the variables.

Notes

Possible values for each variable are ot.OptimizationProblemImplementation.CONTINUOUS, ot.OptimizationProblemImplementation.INTEGER and ot.OptimizationProblemImplementation.`BINARY`.

hasBounds()¶

Test whether bounds had been specified.

Returns:

valuebool: True if bounds had been set for this problem, False otherwise.

hasEqualityConstraint()¶

Test whether equality constraints had been specified.

Returns:

valuebool: True if equality constraints had been set for this problem, False otherwise.

hasInequalityConstraint()¶

Test whether inequality constraints had been specified.

Returns:

valuebool: True if inequality constraints had been set for this problem, False otherwise.

hasLevelFunction()¶

Test whether level function had been specified.

Returns:

valuebool: True if level function had been set for this problem, False otherwise.

hasMultipleObjective()¶

Test whether objective function is a scalar or vector function.

Returns:

valuebool: False if objective function is scalar, True otherwise.

hasResidualFunction()¶

Test whether a least-square problem is defined.

Returns:

valuebool: True if this is a least-squares problem, False otherwise.

isContinuous()¶

Check if the problem is continuous.

Returns:

isContinuousbool: Returns True if all variables are continuous.

isMinimization(marginalIndex=0)¶

Test whether this is a minimization or maximization problem.

Parameters:

marginal_indexint, default=0: Index of the output marginal (for multi-objective only)

Returns:

valuebool: True if this is a minimization problem (default), False otherwise.

setBounds(bounds)¶

Accessor to bounds.

Parameters:

boundsInterval: Problem bounds.

setEqualityConstraint(equalityConstraint)¶

Accessor to equality constraints.

Parameters:

equalityConstraintFunction: Equality constraints.

setInequalityConstraint(inequalityConstraint)¶

Accessor to inequality constraints.

Parameters:

inequalityConstraintFunction: Inequality constraints.

setLevelFunction(levelFunction)¶

Accessor to level function.

Parameters:

levelFunctionFunction: Level function.

setLevelValue(levelValue)¶

Accessor to level value.

Parameters:

levelValuefloat: Level value.

setMinimization(minimization, marginalIndex=0)¶

Tell whether this is a minimization or maximization problem.

Parameters:

minimizationbool: True if this is a minimization problem, False otherwise.
marginal_indexint, default=0: Index of the output marginal (for multi-objective only)

setName(name)¶

Accessor to the object’s name.

Parameters:

namestr: The name of the object.

setObjective(objective)¶

Accessor to objective function.

Parameters:

objectiveFunctionFunction: Objective function.

Notes

Constraints and bounds are cleared if the objective has a different input dimension in order to keep the problem valid at all time.

setResidualFunction(residualFunction)¶

Accessor to the residual function.

Parameters:

residualFunctionFunction: Residual function.

setVariablesType(variableType)¶

Accessor to the variables type.

Parameters:

variablesTypeIndices: Types of the variables.

Notes

Possible values for each variable are ot.OptimizationProblemImplementation.CONTINUOUS, ot.OptimizationProblemImplementation.INTEGER and ot.OptimizationProblemImplementation.BINARY.