LinearEnumerateFunction¶

class LinearEnumerateFunction(*args)¶

Linear enumerate function.

Available constructors:: LinearEnumerateFunction(dim=1)

Parameters:

dimint: Dimension.

See also

EnumerateFunction, HyperbolicAnisotropicEnumerateFunction

Notes

Given an input random vector $\vect{X}$ with prescribed probability density function (PDF) $f_{\vect{X}}(\vect{x})$ , it is possible to build up a polynomial chaos (PC) basis $\{\psi_{\vect{\alpha}},\vect{\alpha} \in \Nset^{n_X}\}$ . Of interest is the definition of enumeration strategies for exploring this basis, i.e. of suitable enumeration functions $\tau$ from $\Nset$ to $\Nset^{n_X}$ , which creates a one-to-one mapping between an integer $j$ and a multi-index $\Nset^{n_X}$ .

Let us first define the total degree of any multi-index $\vect{\alpha}$ in $\Nset^{n_X}$ by $\sum_{i=1}^{n_X} \alpha_i$ . A natural choice to sort the PC basis (i.e. the multi-indices $\vect{\alpha}$ ) is the lexicographical order with a constraint of increasing total degree. Mathematically speaking, a bijective enumeration function $\tau$ is defined by:

$\begin{array}{llcl} \tau \, : & \Nset & \longrightarrow & \Nset^{n_X} \\ & j & \longmapsto & \{\alpha_1,\dots, \alpha_{n_X}\} \, \equiv \, \{\tau_1(j),\dots,\tau_{n_X}(j)\} \\ \end{array}$

such that:

$\tau(0) = \{0,\dots,0\}$

and

$\forall 1 \leq j<k \quad \, , \quad \, \left\{ \begin{array}{l} \displaystyle{\sum_{i=1}^{n_X} \tau_i(j) < \sum_{i=1}^{n_X} \tau_i(k)} \\ \\ \mbox{ or} \\ \\ \displaystyle{\exists \; m \in \{1,\dots,n_X\} \; : \; \left(\forall i \leq m , \; \tau_i(j) = \tau_i(k) \; \right) \, \, \, \mbox{ and } \, \, \, \left(\tau_m(j) < \tau_m(k) \right)} \\ \end{array} \right.$

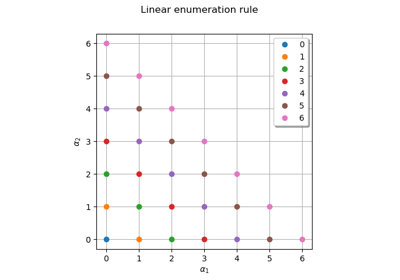
Such an enumeration strategy is illustrated in a two-dimensional case (i.e. $n_X=2$ ) in the figure below:

(Source code, png, hires.png, pdf)

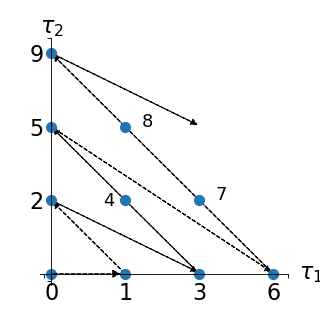
../../_images/LinearEnumerateFunction.png

This corresponds to the following enumeration of the multi-indices:

j	$\vect{\alpha} = \{\alpha_1,\alpha_2\}$
0	{0, 0}
1	{1, 0}
2	{0, 1}
3	{2, 0}
4	{1, 1}
5	{0, 2}
6	{3, 0}
7	{2, 1}
8	{1, 2}
9	{0, 3}

Examples

>>> import openturns as ot
>>> # 4-dimensional case
>>> enumerateFunction = ot.LinearEnumerateFunction(4)
>>> for i in range(9):
...     print(enumerateFunction(i))
[0,0,0,0]
[1,0,0,0]
[0,1,0,0]
[0,0,1,0]
[0,0,0,1]
[2,0,0,0]
[1,1,0,0]
[1,0,1,0]
[1,0,0,1]

Methods

`__call__`(index)	Call self as a function.
`getBasisSizeFromTotalDegree`(maximumDegree)	Get the basis size corresponding to a total degree.
`getClassName`()	Accessor to the object's name.
`getDimension`()	Return the dimension of the EnumerateFunction.
`getId`()	Accessor to the object's id.
`getMaximumDegreeCardinal`(maximumDegree)	Get the number of terms of total degree below a threshold.
`getMaximumDegreeStrataIndex`(maximumDegree)	Get the largest index of the strata containing terms of maximum degree.
`getName`()	Accessor to the object's name.
`getShadowedId`()	Accessor to the object's shadowed id.
`getStrataCardinal`(strataIndex)	Get the number of terms in the basis inside a given strata.
`getStrataCumulatedCardinal`(strataIndex)	Get the number of terms in the basis inside a range of stratas.
`getUpperBound`()	Accessor to the upper bound.
`getVisibility`()	Accessor to the object's visibility state.
`hasName`()	Test if the object is named.
`hasVisibleName`()	Test if the object has a distinguishable name.
`inverse`(indices)	Get the antecedent of a indices list in the EnumerateFunction.
`setDimension`(dimension)	Set the dimension of the EnumerateFunction.
`setName`(name)	Accessor to the object's name.
`setShadowedId`(id)	Accessor to the object's shadowed id.
`setUpperBound`(upperBound)	Accessor to the upper bound.
`setVisibility`(visible)	Accessor to the object's visibility state.

__init__(*args)¶

getBasisSizeFromTotalDegree(maximumDegree)¶

Get the basis size corresponding to a total degree.

Parameters:

max_degint: Maximum total degree.

Returns:

sizeint: Number of terms in the basis of total degree $\leq max_deg$ .

Notes

In the specific context of a linear enumeration (LinearEnumerateFunction) this is also the cumulated cardinal of stratas up to max_deg.

Examples

>>> import openturns as ot
>>> dim = 2
>>> enum_func = ot.LinearEnumerateFunction(dim)
>>> enum_func.getBasisSizeFromTotalDegree(3)
10
>>> enum_func.getStrataCumulatedCardinal(3)
10

getClassName()¶

Accessor to the object’s name.

Returns:

class_namestr: The object class name (object.__class__.__name__).

getDimension()¶

Return the dimension of the EnumerateFunction.

Returns:

dimint, $dim \geq 0$: Dimension of the EnumerateFunction.

getId()¶

Accessor to the object’s id.

Returns:

idint: Internal unique identifier.

getMaximumDegreeCardinal(maximumDegree)¶

Get the number of terms of total degree below a threshold.

Parameters:

max_degint: Maximum total degree.

Returns:

cardinalint: Number of terms in the basis of total degree $\leq max_deg$ .

Notes

In the specific context of a linear enumeration (LinearEnumerateFunction) this is also the cumulated cardinal of stratas of index $\leq max_deg$ .

Examples

>>> import openturns as ot
>>> dim = 2
>>> enum_func = ot.LinearEnumerateFunction(dim)
>>> enum_func.getMaximumDegreeCardinal(2)
6

getMaximumDegreeStrataIndex(maximumDegree)¶

Get the largest index of the strata containing terms of maximum degree.

Parameters:

max_degint: Maximum total degree.

Returns:

indexint: Index of the last strata that contains terms of total degree $\leq max_deg$ .

Notes

In the specific context of a linear enumeration (LinearEnumerateFunction) this is the strata of index max_deg.

Examples

>>> import openturns as ot
>>> dim = 2
>>> enum_func = ot.LinearEnumerateFunction(dim)
>>> enum_func.getMaximumDegreeStrataIndex(2)
2

getName()¶

Accessor to the object’s name.

Returns:

namestr: The name of the object.

getShadowedId()¶

Accessor to the object’s shadowed id.

Returns:

idint: Internal unique identifier.

getStrataCardinal(strataIndex)¶

Get the number of terms in the basis inside a given strata.

Parameters:

strataIndexint: Index of the strata of the tensorized basis.

Returns:

cardinalint: Number of terms in the basis inside the strata strataIndex.

Notes

In the specific context of a linear enumeration (LinearEnumerateFunction) the strata strataIndex consists of a hyperplane of all the terms of total degree strataIndex, and its cardinal is strataIndex + 1.

Examples

>>> import openturns as ot
>>> dim = 2
>>> enum_func = ot.LinearEnumerateFunction(dim)
>>> enum_func.getStrataCardinal(2)
3

getStrataCumulatedCardinal(strataIndex)¶

Get the number of terms in the basis inside a range of stratas.

Parameters:

strataIndexint: Index of the strata of the tensorized basis.

Returns:

cardinalint: Number of terms in the basis inside the stratas of index lower of equal to strataIndex.

Notes

The number of terms is the total of terms inside each consecutive stratas. In the specific context of a linear enumeration (LinearEnumerateFunction) this returns the number of terms of maximal total degree strataIndex.

Examples

>>> import openturns as ot
>>> dim = 2
>>> enum_func = ot.LinearEnumerateFunction(dim)
>>> enum_func.getStrataCumulatedCardinal(2)
6
>>> sum([enum_func.getStrataCardinal(i) for i in range(3)])
6

getUpperBound()¶

Accessor to the upper bound.

Returns:

ubsequence of int: Upper bound of the indices (inclusive).

getVisibility()¶

Accessor to the object’s visibility state.

Returns:

visiblebool: Visibility flag.

hasName()¶

Test if the object is named.

Returns:

hasNamebool: True if the name is not empty.

hasVisibleName()¶

Test if the object has a distinguishable name.

Returns:

hasVisibleNamebool: True if the name is not empty and not the default one.

inverse(indices)¶

Get the antecedent of a indices list in the EnumerateFunction.

Parameters:

multiIndexsequence of int: List of indices.

Returns:

antecedentint: Represents the antecedent of the multiIndex in the EnumerateFunction.

Examples

>>> import openturns as ot
>>> dim = 2
>>> enum_func = ot.LinearEnumerateFunction(dim)
>>> for i in range(6):
...     print(str(i)+' '+str(enum_func(i)))
0 [0,0]
1 [1,0]
2 [0,1]
3 [2,0]
4 [1,1]
5 [0,2]
>>> print(enum_func.inverse([1,1]))
4